WALI BAND BAIK BAIK SAYANG { FULL SONG WITH LYRICS }

WALI BAND MARI SHALAWAT { FULL SONG WITH LYRICS }

Wali Band -Tomat ( Tobat Maksiat )

Wali Band ~ Ya Allah FULL SONG WITH LYRICS

sAlam sumER,,,,,

Huhuuuu,,,,lamer tol ak x tgok blog ak,,,
xda mser tol skang,,,,

Ionic and covalent bonding animation

Chemistry Music Video 16: What Kind Of Bonds Are These?

Chemical Bonding and Atomic Structure (2nd Ed.) (clip)

Percent Composition of Magnesium Oxide Part 2

Balancing Chemical Equations

The Mole and Avogadro's Number

Molecular and Empirical Formulas

Groups of the Periodic Table

Periodic Table Song by Peter Weatherall

They Might Be Giants: "Meet the Elements" (BB Video)

Properties Of Metals And Non- Metals

Transition Metal Experiment 1

Transition Metal Experiment 2

Transition metals.

Physical properties of the halogens

halogen song- chemistry project

Help! The Halogen Song

Halogen Reactions

C001 Chemistry Group 1 elements

Group 1 Metals - Reaction with Water

cHEmiCaL EquAtIoN,,,,

dEDuCtIoN and InDuCTioN.....

ArGUmENt....

ImpLicAtiOn....

OpErAtiOn On sEt....

a) 9 x 9 = 81 or 9 + 9 = 18

b) 3x = -12 when x = -4 or x = -6

c) A quadrilateral whose diagonals bisect at right angles is a square or a rhombus.

d) 25 is divisible by 5 or 10.

chat..chat...chat about math....

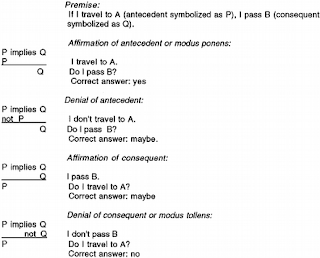
Interesting about mathematical reasoning....

Here, there are so many interesting game.....
So,to all student or everyone view my blog would like to challenge their knowledge,you can do it by complete the following simple game....(cover only about mathematical reasoning)
Thinking about mathematic...... interesting right????

Juz, look at carefully and then answer...make you more creative!!!!

Make a mathematics more interesting in our daily life.....

Hopefully all of you enjoy it....thanks!!!

Math life.....

Ragam housemate ku....

hahaa.....nieh tgn imah.cube tgk die wat per?nk bergmbor,tp jln cm siput....tuh y tertnggal....heheee.sbenarny nk posing y kblkng tuh,tp imah dah cut line r....ciap pkai gelang,comei jri imah....heheeeee

tgk la gayefana tuh, ayu r tuh...heheeee.muker ak,ntah pe2.hahaaa....yg 3 org blkg tuh,posing.nk2 imah....eceh,ciap angkat tgn,lemah lembut r tuh....heheee

hepi kn gmbo ktrng nieh....saper2 tgk pon nk join snyum gak..heheeeee.tp gmbo org tuh jer la y besor.mntang2 die y pgang kamera....cian kt imah kt blkg tuh,nsib bek smpat wat peace....kikihkihkih

yaaaaannnngggg mane saaaaatu.....pilihan mu!!!
heheeee...
imah, aku, zaty, sirin & niniey....
tgk r style imah tuh, da jer kan...huhuuuuu










k....kawan2.Ckup r dlu ragam2 housemateku niey...ti ak amik y pelik2 lgik ea. pastu upload bg korang tgk....hahaaaaa

DuLu Ku sEoRaNG Y GaNAs...heheee

hye sume.....salam.
mule2 dlu ak kadet d PALAPES UPSi,peh byk giler kngn manis & pahit y xkan ak luoekan laa...
dok dlm hutn,jatuh logkang,kena raging,raging org(heeeehee),match netball(johan 3x berturut2),mcm2 laaa....teringat sumer,kt sume pegawai,jurulatih & skuad2 ak tuh...
skang ak dah ayu dah,sopan santun lgik...cheeewahhh.heheeee....dah xkeras pon.
lgipon dah YO(young officer) skang y xaktif nieh...
nek keta kebal mse g Pord dickson...join dak senior mse anual camp....
sweet memories.....(,")

CaMPiNg kt TaSEk bANdiNg....

ewah...pndai dah ak drive boat nieh.
g jln2 ikut kakak keja,menyibuk smbil seronok2...heheeee
kegembiraan y membuat kn diriku t'senyum bile teringat....
heheeee....

sweet memories during practicum session....

zaty, imah,fana,sirin,ninie & me(jue)....
ronggeng2 g taman rekreasi....wekend jer kuar,lepak2...lepaskn tension konon...heheeee
wlaupon xda la stress sgt...
nk2 ckgu STAR n ACS tuh...heheee
hepi giler dok serumah...xkering kusi dok pekena dak sorang tuh...kihkih
hopefully....hepi alwayz my frend.
chaiyok3x!!!!
gambate.....(,")

Diriku insan biase....

nieh kenangan ak g lost world of ambu...be4 ak stat ngajar,release tension coz byk giler masalah timbul during my holiday....(???? terbabas di trafic light)
hahaaaa.....
ak hepi giler g snie sbb mayb 1st time..2nd dpt men2 gn ank buah...heheeee
alhmdlah,everyting lbih baek skang...
thanks god!!!!

Mathematical reasoning(form 4)- Worksheet quantifier.

Let us complete this worksheet....

Worksheet 1 (Quantifier ‘all’ or ‘some’.)

1) Rewrite the following statements by using the quantifier ‘all’ or ‘some’ without
changing the meaning of the statement.

a. Several isosceles triangles are equilateral triangles.
b. Any perfect square is a square of a whole number.
c. Not many of the double digit whole numbers are prime numbers.
d. Every mixed number can be converted to an improper fraction.

2) Determine whether each of the following statements is true or false.

a. All integers can be written as a fraction.
b. Al right-angled triangles have the longest side opposite the right-angled
triangle.
c. All prime numbers do not have factors.
d. All sets produce an empty set when they intersect with an empty set.

3) State whether each statement below is true or false. Give an example to support
your answer.

a. All multiples of 12 are divisible by 4.
b. All cube roots of a negative number is a negative number.
c. All fractions can be written in the form p/q, where p and q are integers
and p is greater than q.
d. Al1 the subsets of the set {2, 3, 4, 5} contain at least on element.

4) Determine whether each of the following statements can be generalized by using
the quantifier ‘all’.

a. A negative integer q gives rise to a positive integer when it is squared.
b. The even number 8 is divisible by 4.
c. Trapezium ABCD has two parallel sides.
d. Pentagon PQRST has five sides.

5) Based on the object and the property given in each of the following, construct a
true statement using the quantifier ‘all’ or ‘some’.

a. Object : Integer.
Property : Whole number.

b. Object : Kites.
Property : One axis of symmetry.

c. Object : Fractions.
Property : Smaller than 1.

d. Object : Right angles.
Property : 900

e. Object : Rectangles.
Property : Equal opposite sides.